-
Homogeneous slow-velocity layer over homogeneous fast-velocity halfspace
- Tested algorithms:
This benchmark case was applied to the following programs: SOFI2D (transformed), SOFI3D, SPECFEM and Gemini
- Scale:
Ultrasonic data
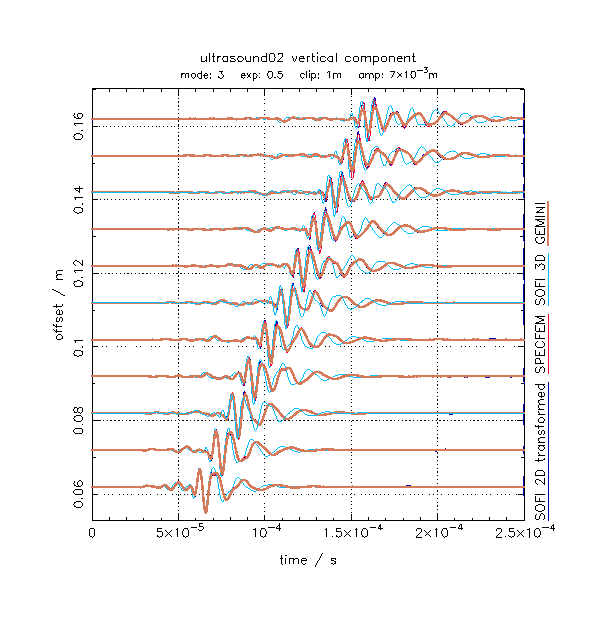
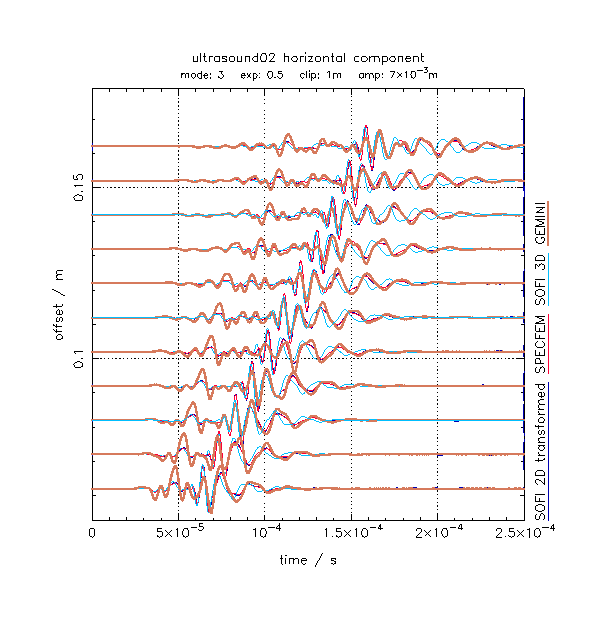
Benchmark case: Homogeneous slow-velocity layer over homogeneous fast-velocity halfspace
Model description
The benchmark model for this case consists of a homogeneous slow-velocity layer (P-wave velocity 2 km/s, S-wave velocity 1.15 km/s and mass density 1.8 g/cm³) over a homogeneous fast-velocity halfspace (P-wave velocity 4 km/s, S-wave velocity 2.31 km/s, mass density 1.8 g/cm³). The layer thickness is 10 mm and elastic wave propagation is assumed. A vertical force source is used as source. Download the model description for a detailed description of the benchmark test including the characteristics of the source and the acquisition geometry.
Compared algorithms
In this benchmark test four different forward solvers are compared: SPECFEM3D, Gemini II, SOFI3D and SOFI2D where results of SOFI2D are transformed to equivalent point-source seismograms. A description of the transformation is provided for download. Please note that proper physical amplitudes of the source are only implemented in SPECFEM3D and SOFI2D.
Time series for download
The calculated time series are provided for download.
The plots displayed on the left and additional zoomed views are provided for download (PDF file). The seismogram displays are produced with refractx. Plot parameters are given in the subtitle of each plot.
The parameter settings used for the plots are given in the label of each plot.
- mode: scaling mode
- 1: scale traces individually
- 2: scale all traces to first trace as reference
- 3: scale all traces to a common offset trace of each dataset as reference
- exp: traces are scaled by offset-dependent factor of (r/1m)exp where r is the offset
- clip: clipping level
- amp: amplitude level
- vred: timescale is reduced by tred=t-r/vred